At What Points is the Function Continuous Calculator
The idea of continuity is simple: If the function graph doesn`t have any breaks or holes inside a positive interval, the function is stated to be non-stop over that interval.
Thus, surely drawing the graph may inform you if the function is non-stop or no longer. However, now no longer all features are smooth to draw, and now and then, we want to apply the definition of continuity to decide a function`s continuity.
Many regions of calculus require information of non-stop features. The examination of non-stop features and discontinuities' residences is an extremely good hobby to the mathematical world. Due to their crucial residences, non-stop features have realistic packages in the system, gaining knowledge of algorithms and optimization processes.
The most common and restrictive definition is that if a function is continuous on all real numbers, then the function is continuous. In this case, the previous two examples are not continuous, but like the sine, cosine, and exponential functions, all polynomial functions are continuous.
The function is right continuous at some point. Here, it can be said that the function of the left endpoint of the interval is continuous if it is continuous to the right there, and the function of the right endpoint of the interval is continuous if it is continuous there.
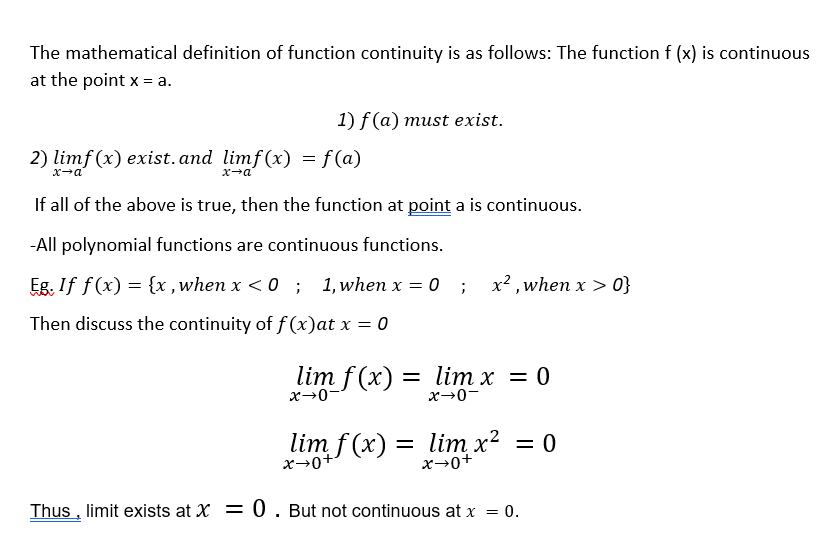
In the analysis, if the curve of the function does not break at the point x = a, then the function f(x) is called a continuous function at the point x = a.
The function f(x) becomes continuous at the point and as x approaches and the value of function f(a) approaches. Therefore, to test the continuity of a function at point x = a, make sure that:
Continuous definition math
A continuous function is a function that has no limits over the entire range or at specific intervals. The graphs do not contain any signs of asymptote or discontinuity. This is a simple continuous definition of math.
Continuous function examples
On the other hand, if one has to consider continuous function examples, a continuous function is a function that can take any number within a certain interval. For example, if a continuous function is 1 at one point and 2 at another point, the continuous function is definitely 1.5 at another point. Continuous functions always connect all their values, but discrete functions have separation.
Continuous function calculator
The continuous function calculator attempts to determine the range, area, x-intersection, y-intersection, the derivative, integral, asymptomatic, interval of increase/decrease, critical (stationary) point, and extremum (minimum and maximum). Local, Relative, Absolute, Global) Search for points—graphs of concave spacing, inflexions, limits, Taylor polynomials, and one-variable functions. You can specify the interval. Parity is also determined.
Properties of continuous functions
The Intermediate Value Theorem. If a function is continuous on an interval, and it takes on two values in that interval, then it takes on all intermediate values. Symbolically, if f is continuous on [a,b], and y is between f(a) and f(b), there there is some x in [a,b] such that f(x) = y.
The Extremal Value Theorem. If a function is continuous on a closed interval, then it takes on a maximum value and a minimum value. Symbolically, if f is continuous on [a,b], then there is some c in [a,b] such that f(c) ≥ f(x) for all x in [a,b]. Likewise, there is some d in [a,b] such that f(d) ≤ f(x) for all x in [a,b].
The sum, difference, and product of two continuous functions are each continuous function. All polynomial functions are continuous.
The quotient of two continuous functions is continuous where it is defined. (It won't be defined when the denominator is zero.) All rational functions (quotients of two polynomials) are continuous where they're defined.
Conclusion
Continuous functions are very crucial while investigating optimization problems. We can see that the extremum theorem ensures that a factor continually exists withinside the c program language period in which the function has the most value.
The equal is proper for the minimal value. Many optimization algorithms derive from this primary function and may carry out great tasks.
Source: https://unacademy.com/content/cbse-class-12/study-material/mathematics/continuous-functions/
0 Response to "At What Points is the Function Continuous Calculator"
Post a Comment